Superposition Theorem Ppt For Mac

1. Superposition Theorem Pdf
2. Superposition Theorem Definition

Download Presentation PowerPoint Slideshow about 'Thevenin’s theorem, Norton’s theorem and Superposition theorem for AC Circuits' - vachel An Image/Link below is provided (as is) to download presentation Download Policy: Content on the Website is provided to you AS IS for your information and personal use and may not be sold / licensed / shared on other websites without getting consent from its author.While downloading, if for some reason you are not able to download a presentation, the publisher may have deleted the file from their server.

Superposition Theorem Pdf

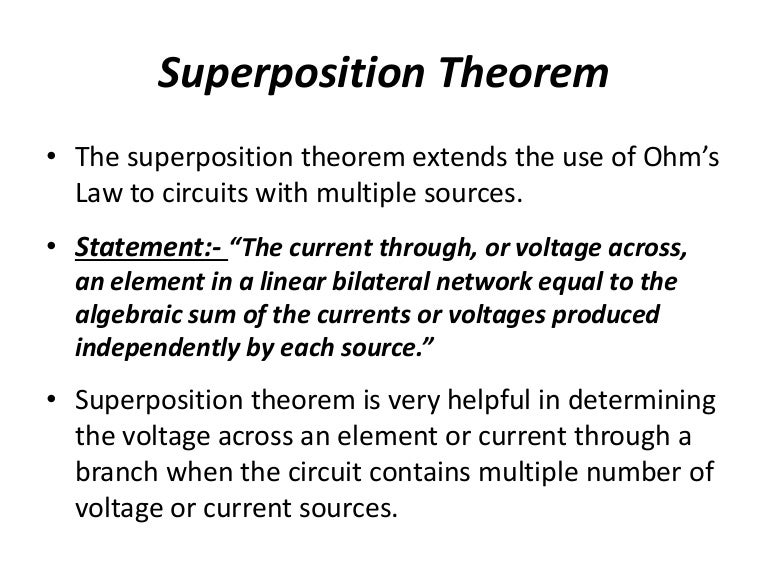
Superposition Theorem Definition

View Superposition Theorem presentations online, safely and virus-free! Many are downloadable. Learn new and interesting things. Get ideas for your own presentations. Share yours for free! SUPERPOSITION THEOREM SOLVED PROBLEMS PPT - In this site isn`t the same as a. Revise this using your PC, MAC, tablet, eBook reader or smartphone. Superposition Theorem (2/2) If Dependent Sources Exist, They Must Remain In PPT. Presentation Summary: Superposition Theorem (2/2) If dependent sources exist, they must remain in the circuit for each solution. Nonlinear responses such as power cannot be found.

Contents. Introduction The Principle of Superposition is a method used to solve complex problems with multiple loads and/or reactions acting on the member. Superposition helps us solve these problems by breaking the member down as many times as necessary for each force acting on it. Once all the stresses or deflections for the point of interest are found, they can then be added all together to get a final answer.

Important Terms Deflection: The deviation of a point of interest, from it's original position, due to an external force acting on the member. Distributed Load: Uniform external forces that acts on the surface of a member over a specific length. External Load: Are the forces acting on the surface of a member. These can include support reactions,applied forces, normal force etc.

Hooke's Law of Elasticity: For relatively small deformations of an object, the displacement or size of the deformation is directly proportional to the deforming force or load. Internal Load: Are the forces that act on a member from the inside. It is the forces that hold the member together when external forces are being applied.

Point Load: Is a force acting on a single stationary point on a given structure. Summary 'The principle of superposition simply states that on a linear elastic structure, the combined effect of several loads acting simultaneously is equal to the algebraic sum of the effects of each load acting individually.' Hibbeler states this as 'The total displacement or internal loadings (stress) at a point in a structure subjected to several external loads, which can be determined by adding together the displacements or internal loadings (stress) caused by each external load acting separately.' In other words, for a linearly elastic structure, the effect of several loads acting on a member is equal to the summation of the loads acting separately.

Figure 1: Principle of Superposition Simplified usage of Superposition shown in FIGURE 1: A given beam and its loadings can be split into simpler beams and loading. As shown in figure 1, the beam with the distributed load and the point load can be split into two beams.

One beam with the distributed load and the other with the point load. Conditions There are two conditions in which a structure must satisfy in order for the principle to be valid. Structures which do satisfy these two conditions are referred to as Linearly Elastic Structures. The two conditions are as follows: i. The equations of equilibrium must be based off of the undeformed shape of the structure: If the size of the deformations occurring to the structure are small enough to be considered negligible, it can be determined that the undeformed shape of the structure can be used as a basis for the equilibrium equations. The material used in the structure must be Linearly Elastic: In order for a material to be considered this way, It's stress-strain relationship must relate to Hooke's Law of elasticity, referring to the stiffness of the materials. Equation The right side of the equation is the algebraic sum of the left side of the equation.

$ f(z1 + z2 + z3 +. + zn) = f(z1) + f(z2) + f(z3)+. + f(zn) $ Sign Convention In order to prevent confusion and maintain clarity throughout a complex analysis, an arbitrary axis system is established and positive directions indicate tensional or compressive forces, whichever is established.

Negative values in a positive tension orientation would indicate a compressive force and a negative force in a positive compression orientation would indicate a tensional force. Typically, an X-Y coordinate system would be labelled upwards and rightwards with tension being represented as a positive structural force. Thus any negative force intuitively is compressive. Trigonometry Since Superposition states that complex plane forces can be analyzed by respective axis’s, how the forces are decomposed is important and must follow convention. To break these directionally-complex forces into simple axis forces, trigonometry must be extensively used. Any force acting on a support structure acts on some direction to the beam which is supporting it.

The angle between the acting force and the plane of the beam is typically used as the basis angle to which the angle acting on the beam will be broken down into parallel and perpendicular components of the force acting on the beam. Application There are many different ways that superposition can be used to analyze structures. Listed below are a couple of applications that it can be used for. The application of the principle of superposition in the method of sections is applied to a section of a frame and rather than analyzing a single joint at a time, a selection of members are cut and equilibrium is established using the internal forces of the cut members. Superposition is used to determine internal forces of the cut member by the equilibrium equations where; $ Sigma Fx = 0, $ $ Sigma Fy = 0, $ $ Sigma M = 0 $. Where $ Sigma Fx = 0 $ means the total forces (external and internal) that lie in the x-axis is equal to zero.

$ Sigma Fy = 0 $ is the total forces (external and internal) that lie in the y-axis is equal to zero. $ Sigma Mp = 0 $ is the the total moment about point p is also equal to zero. Equilibrium and Superposition Since the principle of superposition states that 'the resultant stress or displacement (or force) can be determined by algebraically summing the stress or displacement (or force) caused by each load component applied separately to the member'. With equilibrium stated as a balance of forces, the two can be equated mathematically as follows: Superposition: $ Sigma F = Sigma Fn $ $ Sigma M = Sigma Mn $ Equilibrium: $ Sigma F = 0 $ $ Sigma M = 0 $ Therefore we can see that: $ Sigma F = Sigma Fn = 0 $ $ Sigma M = Sigma Mn = 0 $ Which means that for a given static system with applied external loads, the total sum of the forces acting on the member(s) is the applied external forces plus the reaction forces or moments.

Example Given Figure 2, use superposition to find the resulting deformation at point E due to the loads applied. $ E=250 $ $ GPa $ and $ I = 125 times 10^6 mm $.

Figure 3: Free Body Diagram First we will use our equilibrium equations to find our support reactions. $ Sigma M = 0 $ $ 0 = -50 (10) + Cy (20) - 12 cos 45 (25) - 75 $ $ Cy = 39.4 kN $ $ Sigma Fy = 0 $ $ 0 = -50 +39.4 - 12 cos 45 +Ay $ $ Ay = 19.1 kN $ $ Sigma Fx = 0 $ $ 0 = Ax - 12 cos 45 $ $ Ax = 8.5 kN $ We can now see our converted beam which is ready for our superposition method. Now we can solve our individual deflections due to each force acting on the member.

We know with our second moment area theorem that.